
Infringement Notice, it will make a good faith attempt to contact the party that made such content available by If Varsity Tutors takes action in response to Information described below to the designated agent listed below. Or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one This is the final answer, which is an approximation of the area under the function. Now that we have all the pieces, we can plug them in. To find the next endpoint,, just increase the first x by the length of the subinterval, which is. In other words, since we only care about the area from to, we'll just use the smaller one,, for our first. The left most x value happens to be the smaller of the overall endpoints given in the question. Left endpoints because we are doing Left Riemann sums. Now we need to find the x values that are the left endpoints of each of the 4 subintervals. We then split this total length into 4 pieces, since we are told to use 4 subintervals. To find we find the total length between the beginning and ending x values, which are given in the problem as and.
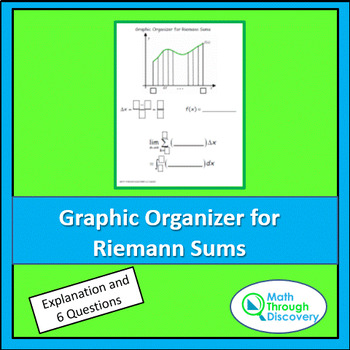
This is basically, 4 times, and then added together.


Think of as the base of each box, and as the height of the 1st box. We can rewrite this fancy equation by writing, 4 times 1 time each for, ,, and. This fancy equation approximates using boxes. , is the width of each subinterval, which we will determine shortly.Īnd means add all versions together (for us that means add up 4 versions). Is the function value when you plug in the "i-th" x value, (i-th in this case will be 1-st, 2-nd, 3-rd, and 4-th) Is the "counter" that denotes which subinterval we are working with,(4 subintervals mean that will be 1, 2, 3, and then 4) Where is the number of subintervals, (4 in our problem), To use left Riemann sums, we need to use the following formula:


 0 kommentar(er)
0 kommentar(er)
